Day 25 - Resonance

---

Announcements

• Midterm 1 is still being graded

• Homework 6 is due Friday

• Homework 7 is posted, due next Friday

• No office hours today

---

Seminars this week

Most of MSU folks are at APS Global Physics Summit

WEDNESDAY, March 19, 2025

• Astronomy Seminar, 1:30 pm, 1400 BPS, Alex Rodriguez, University of Michigan, Galaxy clusters, cosmology, and velocity dispersion

• FRIB Nuclear Science Seminar, 3:30pm., FRIB 1300 Auditorium, Dr. Pierre Morfouace of CEA-DAM, Mapping the new asymmetric fission island with the R3B/SOFIA setup

THURSDAY, March 20, 2025

Colloquium, 3:30 pm, 1415 BPS, Guillaume Pignol, University of Grenoble, Ultracold neutrons: a precision tool in fundamental physics

---

Reminders

We started to solve the forced harmonic oscillator equation:

\[\ddot{x} + 2 \beta \dot{x} + \omega_0^2 x = f(t)\]

We examined the case of a sinusoidal driving force:

\[\ddot{x} + 2 \beta \dot{x} + \omega_0^2 x = f_0 \cos(\omega t)\]

There’s a complimentary case where the driving force is a sine wave:

\[\ddot{y} + 2 \beta \dot{y} + \omega_0^2 y = f_0 \sin(\omega t)\]

---

Reminders

We combined the two equations into a complex equation using these identities:

\[z(t) = x(t) + i y(t)\]

\[e^{i \omega t} = \cos(\omega t) + i \sin(\omega t)\]

The resulting equation is:

\[\ddot{z} + 2 \beta \dot{z} + \omega_0^2 z = f_0 e^{i \omega t}\]

Notice that there’s a homogeneous part (\(z_h\)) and a particular part (\(z_p\)).

\[\ddot{z}_h + 2 \beta \dot{z}_h + \omega_0^2 z_h = 0\]

---

Reminders

The homogeneous part is the solution we’ve found before with the general solution:

\[z_h(t) = C_1 e^{rt} + C_2 e^{r^* t}\]

where \(r = -\beta \pm i \sqrt{\omega_0^2 - \beta^2}\). In the case of a weakly damped oscillator (\(\beta^2 < \omega_0^2\)), we have:

\[z_h(t) = e^{-\beta t} \left( C_1 e^{-i \sqrt{\omega_0^2 - \beta^2} t} + C_2 e^{+i \sqrt{\omega_0^2 - \beta^2} t} \right)\]

These solutions die out as \(t \to \infty\). They are called transient solutions.

---

Solving the particular part

The particular part is the solution to the driven harmonic oscillator equation:

\[\ddot{z}_p + 2 \beta \dot{z}_p + \omega_0^2 z_p = f_0 e^{i \omega t}\]

Assume a sinusoidal solution (frequency, \(\omega\)) of the form: $\(z_p(t) = C e^{i \omega t}\)$

where \(C\) is a complex number. Then, we have:

\[-\omega^2 C e^{i \omega t} + 2 i \beta \omega C e^{i \omega t} + \omega_0^2 C e^{i \omega t} = f_0 e^{i \omega t}\]

\[\left(-\omega^2 + 2 i \beta \omega + \omega_0^2\right) C = f_0\]

---

Amplitude of the particular solution

\[C =\dfrac{f_0}{\left(\omega_0^2-\omega^2 + 2 i \beta \omega\right)}\]

We want to convert this to polar form:

\[C = A e^{-i \delta}\]

where \(A\) and \(\delta\) are real numbers. We use the complex form to compute the magnitude of the amplitude:

\[A^2 = C \bar{C} = \dfrac{f_0^2}{\left(\omega_0^2-\omega^2 + 2 i \beta \omega \right)\left(\omega_0^2-\omega^2 - 2 i \beta \omega \right)}\]

\[A^2 = \dfrac{f_0^2}{(\omega_0^2 - \omega^2)^2 + 4 \beta^2 \omega^2}\]

---

Clicker Question 24-5

We found that the square amplitude of the driven harmonic oscillator is:

\[A^2 = \dfrac{f_0^2}{(\omega_0^2 - \omega^2)^2 + 4 \beta^2 \omega^2}\]

When is the amplitude of the driven oscillator maximized?

1. When the driving frequency (\(\omega\)) is far from the natural frequency (\(\omega_0\))

2. When the driving frequency (\(\omega\)) is close to the natural frequency (\(\omega_0\))

3. When the damping (\(2\beta\)) is weak

4. When the damping (\(2\beta\)) is strong

5. Some combination of the above

---

Finding the phase

With,

\[C =\dfrac{f_0}{\left(\omega_0^2-\omega^2 + 2 i \beta \omega \right)} = Ae^{-i\delta}\]

then we can compare the complex forms:

\[f_0 e^{i \delta} = A \left(\omega_0^2-\omega^2 + 2 i \beta \omega \right).\]

Both \(f_0\) and \(A\) are real numbers, so the phase \(\delta\) is the same phase as the complex number:

\[\delta = \tan^{-1}\left(\dfrac{2 \beta \omega}{\omega_0^2 - \omega^2}\right)\]

---

The Particular Solution

Let’s return to the particular solution:

\[z_p(t) = C e^{i \omega t} = A e^{-i \delta} e^{i \omega t} = A e^{i(\omega t - \delta)}\]

\[z_p(t) = A \cos(\omega t - \delta) + i A \sin(\omega t - \delta)\]

So we get solutions to both driven oscillators:

\[x_p(t) = Re(z_p(t)) = A \cos(\omega t - \delta)\]

\[y_p(t) = Im(z_p(t)) = A \sin(\omega t - \delta)\]

These are the steady-state solutions.

They persist as \(t \to \infty\) and oscillate at the driving frequency \(\omega\).

---

The Full Solution

\[x(t) = x_h(t) + x_p(t)\]

Here, \(x_h(t)\) is the transient solution and \(x_p(t)\) is the steady-state solution.

For weakly damped oscillators, the transient solution can be written in the form:

\[x_h(t) = A_{tr}e^{-\beta t} \cos(\sqrt{\omega_0^2 - \beta^2} t + \delta_{tr})\]

where \(A_{tr}\) and \(\delta_{tr}\) are real numbers and are the amplitude and phase of the transient solution. Both are determined by the initial conditions.

\[x_p(t) = A \cos(\omega t - \delta)\]

where \(A\) and \(\delta\) are real numbers and are the amplitude and phase of the steady-state solution.

---

The Full Solution

The transient plus the steady-state solution is the full solution:

\[x(t) = A_{tr}e^{-\beta t} \cos(\sqrt{\omega_0^2 - \beta^2} t + \delta_{tr}) + A \cos(\omega t - \delta)\]

As \(t \to \infty\), the transient solution dies out and the steady-state solution persists.

\[x(t \to \infty) = A \cos(\omega t - \delta)\]

where

\[A = \dfrac{f_0}{\sqrt{(\omega_0^2 - \omega^2)^2 + 4 \beta^2 \omega^2}}\]

\[\delta = \tan^{-1}\left(\dfrac{2 \beta \omega}{\omega_0^2 - \omega^2}\right)\]

---

Resonance

The amplitude of the steady-state solution is:

\[A^2 = \dfrac{f_0^2}{(\omega_0^2 - \omega^2)^2 + 4 \beta^2 \omega^2}\]

We change \(\omega_0\) and observe how the amplitude changes.

---

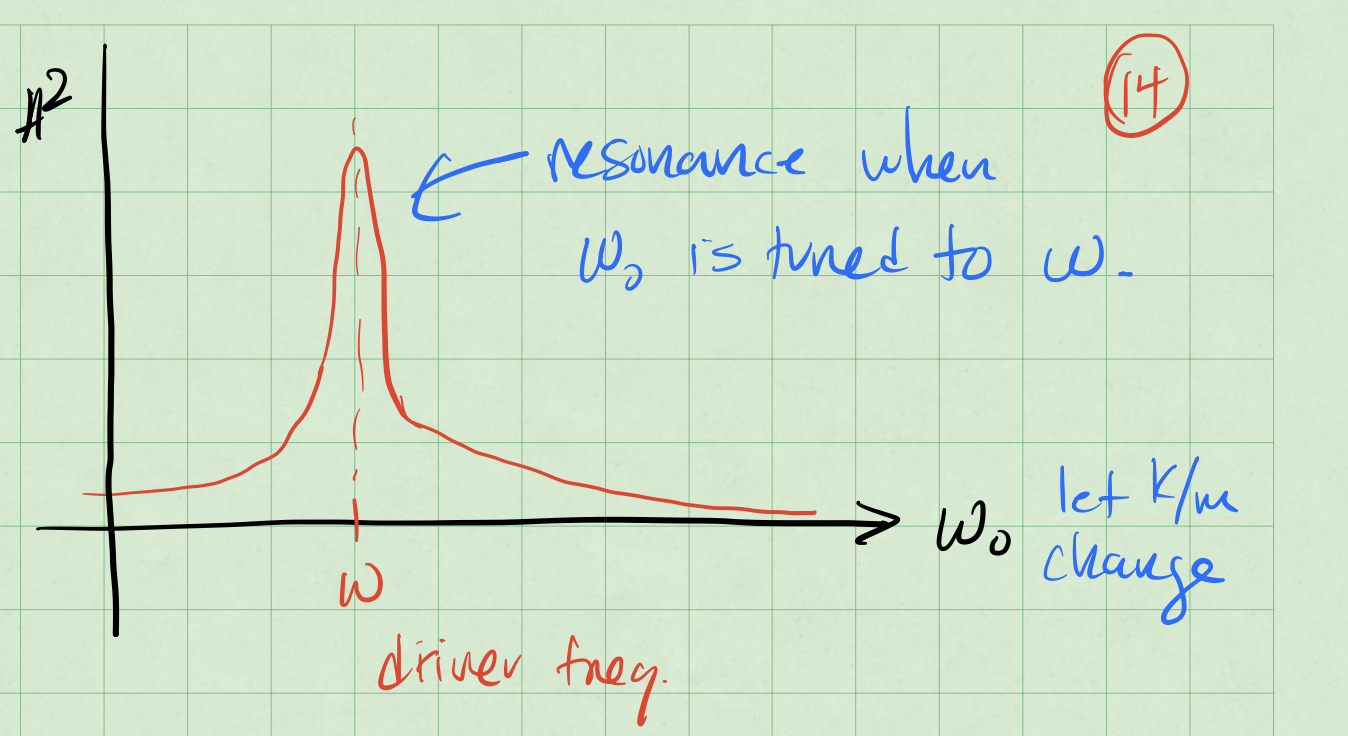
Achieving resonance

The denominator of the equation controls the amplitude:

\[(\omega_0^2 - \omega^2)^2 + 4 \beta^2 \omega^2\]

Case 1: Tune \(\omega_0\) to be close to \(\omega\). Car Radio tuning

With \(\omega_0 = \omega\), the amplitude is:

\[A^2 = \dfrac{f_0^2}{4 \beta^2 \omega^2}\]

---

Achieving resonance

The denominator of the equation controls the amplitude:

\[(\omega_0^2 - \omega^2)^2 + 4 \beta^2 \omega^2\]

Case 2: Tune \(\omega\) to be close to \(\omega_0\). Pushing a swing

Find the \(\omega\) that maximizes the amplitude by taking the derivative with respect to \(\omega\):

\[\dfrac{d}{d\omega} \left((\omega_0^2 - \omega^2)^2 + 4 \beta^2 \omega^2\right) = 0\]

\[2(\omega_0^2 - \omega^2)(-2\omega) + 8 \beta^2 \omega = 0\]

\[4\omega(\omega^2 - \omega_0^2 + 2 \beta^2) = 0\]

\[\omega = 0 \qquad \omega = \sqrt{\omega_0^2 - 2 \beta^2}\]