Day 07 - Drag Forces

Day 07 - Drag Forces#

bg right width:100%

Big Announcement#

MSU Tenure System Faculty Union Recognized#

What does that mean for you?#

  • Faculty can bargain with the university for what’s needed to meet our educational needs

  • For example, there’s more of y’all every year, but not more of us. bg right 90%

Announcements#

  • Homework 2 is due Friday; late after Sunday 11:59pm (always)

  • Homework 3 is now posted; due next Friday; late after Sunday…

  • Office hours, for now (Mihir-MN; Danny-DC):

    • Tuesday 6-8pm (MN, Zoom)

    • Thursday 6-8pm (MN, Zoom)

    • Friday 2-4pm (DC, 1248 BPS)

  • Zoom Link: https://msu.zoom.us/j/96882248075

    • password: phy321msu

  • Friday’s Class: Will work Exercises 3a, 5a, & 5b form HW 3 together + questions

Seminars this week#

WEDNESDAY, September 10, 2025#

Astronomy Seminar, 1:30 pm, 1400 BPS, In Person and Zoom, Host~ Speaker: Rachael Roettenbacher, University of Michigan Title: Imaging Spotted Stars for an Improved Understanding of Stars and Exoplanets Zoom Link: https://msu.zoom.us/j/887295421?pwd=N1NFb0tVU29JL2FFSkk0cStpanR3UT09 Meeting ID: 887-295-421 Passcode: 002454

Seminars this week#

WEDNESDAY, September 10, 2025#

FRIB Nuclear Science Seminar, 3:30pm., FRIB 1300 Auditorium and online via Zoom Speaker: Suzanne Lapi of the University of Alabama at Birmingham Title: Development of new isotopes for theranostic applications Please see website for full abstract. Please click the link below to join the webinar: Join Zoom: https://msu.zoom.us/j/96485010083?pwd=O0rXwspn80aYGEI06QEZag6Ao4siq7.1 Meeting ID: 964 8501 0083 Passcode: 261744

Seminars this week#

THURSDAY, September 11, 2025#

Colloquium, 3:30 pm, 1415 BPS, in person and zoom. Host ~ Refreshments and social half-hour in BPS 1400 starting at 3 pm Speaker: Laura Chomiuk, MSU Title: Fall 2025 Physics and Astronomy Kickoff Background: For more information and to schedule time with the speaker, see the colloquium calendar at https://pa.msu.edu/news-events-seminars/colloquium-schedule.aspx Zoom Link: https://msu.zoom.us/j/94951062663 Password: 2002 Or complete link: https://msu.zoom.us/j/94951062663?pwd=c48uM25P9UsRVuR74rkOioOWgpoxgC.1

Seminars this week#

FRIDAY, September 12, 2025#

QuIC Seminar, 12:30pm, -1:30pm, 1300 BPS, In Person
Speaker: Jean Paul Sadia, MSU Title: Introduction to Quantum Information and Computation Full Scheule is at: https://sites.google.com/msu.edu/quic-seminar/ For more information, reach out to Ryan LaRose

Seminars this week#

FRIDAY, September 12, 2025#

IReNA Online Seminar, 2:00 pm, via Zoom. Hosted by: Artemis Tsantiri (University of Regina, Canada) Speaker: Lorenzo Roberti, INFN-LNS, Italy/Konkoly, Observatory Hungary Title: Carbon-Oxygen Shell Mergers in Massive Stars Zoom Link: https://msu.zoom.us/j/827950260 Password: JINA

AI Policy Proposals#

  • Proposal 1: We adopt a policy that does not allow AI use at all.

  • Proposal 2: We adopt a policy that allows AI use for brainstorming, help, and editing.

  • Proposal 3: We adopt a policy that allows AI for use in nearly any way.

  • Proposal 4: We adopt a policy that allows AI for use in any way with no documentation required.

Updated AI Policy#

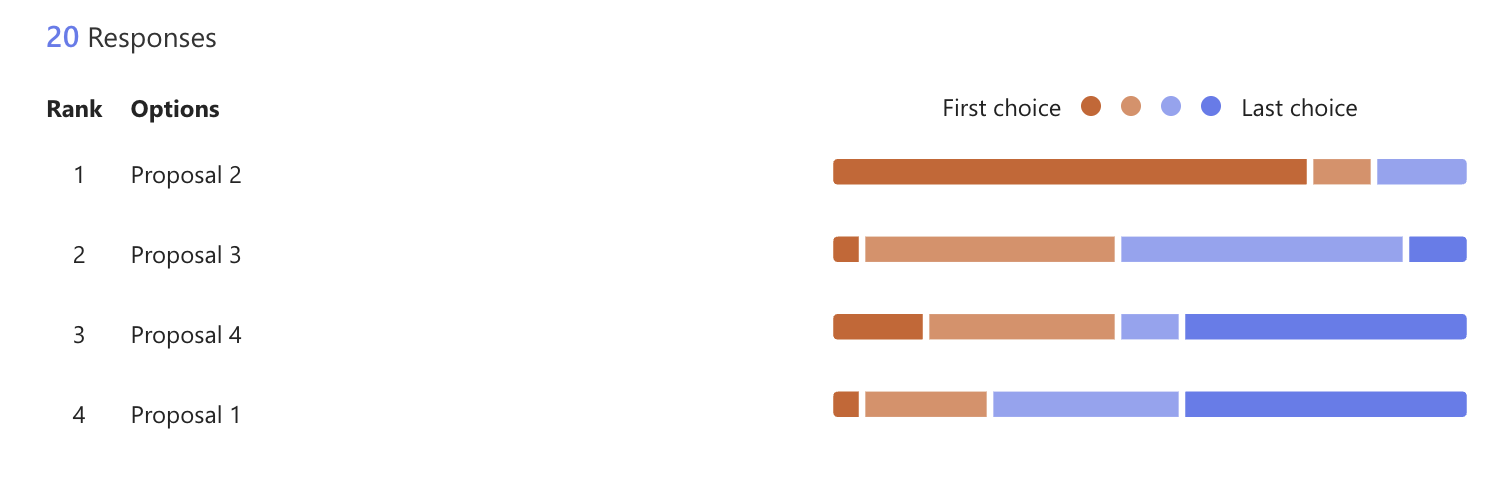
Proposal 2#

We adopt a policy that allows AI use for brainstorming, help, and editing.

  • AI cannot be used for direct answers or completion of assignments.

  • We expect documentation of AI use, but it can be informal.

  • Violations are discussed with Danny; the first violation requires a redo of the assignment, and repeated violations result in a failing grade.

Received 75% of first choice votes

Goals for Week 3#

  • Be able to answer the following questions.

    • What is Mathematical Modeling?

    • What is the process for analyzing these models?

  • Be able to solve “Simple” Motion Problems with Newton’s Laws.

Our man, Reynolds#

bg right width:300px

  • The Reynolds number is a dimensionless quantity.

  • It is a ratio of inertial forces to viscous forces.

\[Re = \frac{\rho v L}{\mu}\]

  • \(\rho\) - density of the fluid

  • \(v\) - velocity of the object

  • \(L\) - characteristic length

  • \(\mu\) is the dynamic viscosity

Our man, Reynolds#

bg right width:300px

BTW, this is not a photo of Reynolds.

  • This is Stokes.

    • He developed the concept of the Reynolds number.

    • Reynolds “popularized” it according to the Wikipedia.

\[Re = \frac{\rho v L}{\mu}\]

Discussion: What kinds of systems have a high/low Reynolds number?

Clicker Question 6-2a#

I made a mistake explaining our linear drag model derivation.

Consider a ball falling down with a coordinate system such that \(y\) is positive downward (as before). Which of the following is correct for the differential equation:

  1. \(m\ddot{y}=-mg+bv_y\)

  2. \(m\ddot{y}=-mg-bv_y\)

  3. \(m\ddot{y}=+mg+bv_y\)

  4. \(m\ddot{y}=+mg-bv_y\)

Think about the SIGN of \(v_y\); it’s a component not a speed!

Clicker Question 6-2b#

Assuming a linear model for Air Resistance \(\sim bv\), we can obtain this EOM for the velocity a falling ball, after separation of variables:

\[\dfrac{dv_y}{v_y - v_{term}} = -\frac{b}{m}dt\]

Assume we try to integrate this equation from \(v_y = v_{y0}\) at \(t=0\) to \(v_y(t)\) at time \(t\).

Write the integral (on both sides) that is needed to solve this equation.

This integral setup is common in physics derivations with ODEs; provided we can find an anti-derivative.

Clicker Question 6-3#

For the system of Linear Drag in 1D, we found a solution for the velocity as a function of time, with \(v = 0\) at \(t = 0\). $\(v(t) = v_{term}\left(1-e^{-\dfrac{bt}{m}}\right)\)$

where \(v_{term} = \sqrt{\frac{mg}{b}}\).

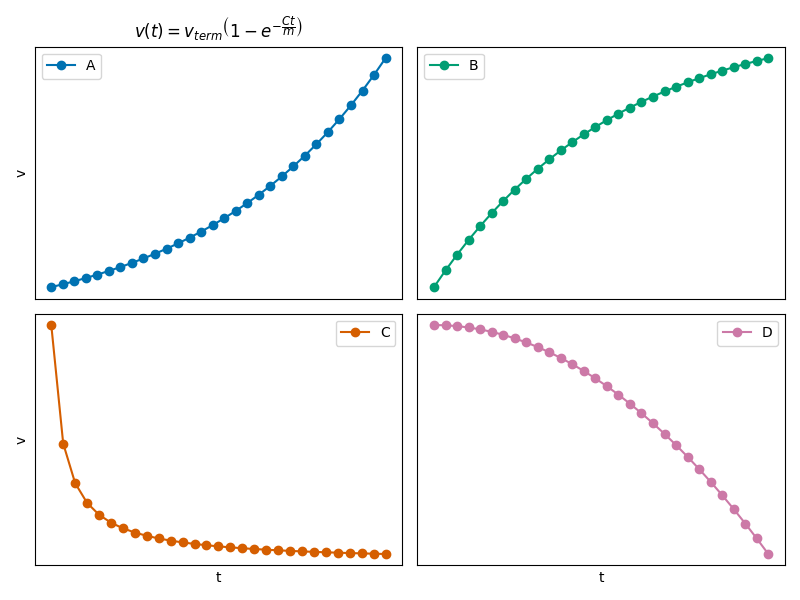
bg right w:100%

CQ 6-3#

Which sketch could be correct for the velocity of the ball?

Clicker Question 6-4#

For the system of Quadratic Drag in 1D, we found a solution for the velocity as a function of time, with \(v = 0\) at \(t = 0\).

\[v(t) = v_{term}\tanh(gt/v_{term})\]

where \(v_{term} = (mg/c)^{1/2}\). Do the units make sense? What are the units \(\left[gt/v_{term}\right]\)?

  1. Yes,\(v\) and \((mg/c)^{1/2}\) have the same units; the units for \(\left[gt/v_{term}\right]\) are m/s.

  2. No, \(v\) and \((mg/c)^{1/2}\) have different units; the units for \(\left[gt/v_{term}\right]\) are m/s.

  3. Yes, \(v\) and \((mg/c)^{1/2}\) have the same units; the units for \(\left[gt/v_{term}\right]\) are unit-less.

  4. No, \(v\) and \((mg/c)^{1/2}\) have different units; the units for \(\left[gt/v_{term}\right]\) are unit-less.

Clicker Question 6-5#

For the system of Quadratic Drag in 1D, we found a solution for the velocity as a function of time, with \(v = 0\) at \(t = 0\).

\[v(t) = v_{term}\tanh(gt/v_{term})\]

where \(v_{term} = \sqrt{mg/c}\). What happens when \(t \rightarrow \infty\)?

  1. The object stops moving.

  2. The object travels at a constant velocity.

  3. The object travels at an increasing velocity.

  4. The object travels at a decreasing velocity.

  5. I’m not sure.

Contents